
développement limité fonction plusieurs variables
Cet outil vous permettra de calculer le développement d'une fonction jusqu'à l'ordre 10 . Vous avez juste à renseigner la fonction voulue et en quel point vous voulez effectuer le développement limité. Le développement limité ainsi que sa représentation graphique sera affiché ci-dessous. Veuillez saisir la fonction f (x)
Concours PUISSANCE ALPHA 2014 Mathématiques (Corrigé) AlloSchool
Alpha Xi Delta National Housing Corporation Inc, Building Corporation Of St Louis Mo: Employer Identification Number (EIN) 237444685: Name of Organization: Alpha Xi Delta National Housing Corporation Inc: Secondary Name: Building Corporation Of St Louis Mo: In Care of Name: Kim Mulder Pres: Address: 1149 Arbor Cr Dr 3d, Kirkwood, MO 63122.

Concours PUISSANCE ALPHA 2013 Mathématiques (Corrigé) AlloSchool
TeX. \alpha. SVG. Download ↓. All symbols. The 'α' is a letter of the Greek alphabet. In mathematics, physics, and engineering, it is often used to denote an angle, a coefficient of thermal expansion, as an alpha particle, among other uses. The 'α' is a letter of the Greek alphabet.
Concours PUISSANCE ALPHA 2012 Physique (Sujet) AlloSchool
Sens de variation : croissante si $\alpha>1$, décroissante si $\alpha1$, constante si $\alpha=0$. Limites aux bornes : si $\alpha>0$, alors $\lim_{x\to 0}x^\alpha=0$ et $\lim_{x\to+\infty}x^\alpha=+\infty$;

Développement limité avec une puissance YouTube
lim x → 0 ε ( x) = lim x → 0 x 1 + x = 0. La fonction f ( x) = 1 1 + x admet alors un développement limité en 0 à l'ordre n. Par le théorème d'unicité du développement limité, on a bien le résultat: 1 1 + x = 1 − x + x 2 + ⋯ + ( − 1) n x n + o ( x n) Si vous avez trouvé cet article ou ce site utile et souhaitez soutenir.

Concours PUISSANCE ALPHA 2013 Chimie (Sujet) AlloSchool
Futura-Sciences : les forums de la science. MATHEMATIQUES. Mathématiques du supérieur. Développement limité puissance X. Affichage des résultats 1 à 5 sur 5.
Concours PUISSANCE ALPHA 2016 Mathématiques (Sujet) AlloSchool
The Liver and Transplant Center at St. Louis Children's Hospital provides diagnosis and treatment options for children with alpha-1 antitrypsin deficiency. For more information or to schedule an appointment, call 314.454.5437 or 800.678.5437 or email us. Pediatric Liver Care and Transplant Center.
Concours PUISSANCE ALPHA 2013 Mathématiques (Corrigé) AlloSchool
Pour calculer un développement limité (DL) d'ordre n d'une fonction f(x) au voisinage d'une valeur a, si la fonction est dérivable en a, alors il est possible d'utiliser la formule de Taylor-Young qui décompose toute fonction en : f(x) = f(a) + f (a) 1! (x − a) + f ( 2) (a) 2! (x − a)2 + ⋯ + f ( n) (a) n! (x − a)n + O(xn + 1) = n.

Concours PUISSANCE ALPHA 2015 Physique (Sujet) AlloSchool
19-Nor-1-alpha-25-dihydroxyvitamin D2 (Paricalcitol) safely and effectively reduces the levels of intact parathyroid hormone in patients on hemodialysis. normalized serum calcium levels between 8.0 and 10.0 mg/dl, and calcium x phosphorus product values less than 75. Study end points included a decrease in iPTH of at least 30% or a maximum.
Formulaires disponibles Développement limité fiche méthode
Alpha Xi Delta Fraternity: Secondary Name: Epsilon Xi Chapter: In Care of Name: University Of Missouri St Louis: Address: 8001 Natural Bridge Rd, St Louis, MO 63121-4401:. You can rate this nonprofit in different categories from 1 star (worst) to 5 stars (best) or leave category unrated. Program: Fundraising: Expenses: Transparency:

Concours PUISSANCE ALPHA 2017 Biologie (Corrigé) AlloSchool
Maths L1 AnalyseDans cette vidéo on calcule le développement limité de (1+x)^alpha en utilisant la formule de Taylor YoungNiveau licence scientifique 1ère an.
Concours PUISSANCE ALPHA 2016 PhysiqueChimie (Sujet) AlloSchool
lim x → 0 ε ( x) = lim x → 0 − x 1 − x = 0. La fonction f ( x) = 1 1 − x admet alors un développement limité en 0 à l'ordre n. Par le théorème d'unicité du développement limité, on a bien le résultat: 1 1 − x = 1 + x + ⋯ + x n + o ( x n) Si vous avez trouvé cet article ou ce site utile et souhaitez soutenir notre.
Concours Puissance Alpha 1 Puissance Alpha Concours J'ai 20 en maths
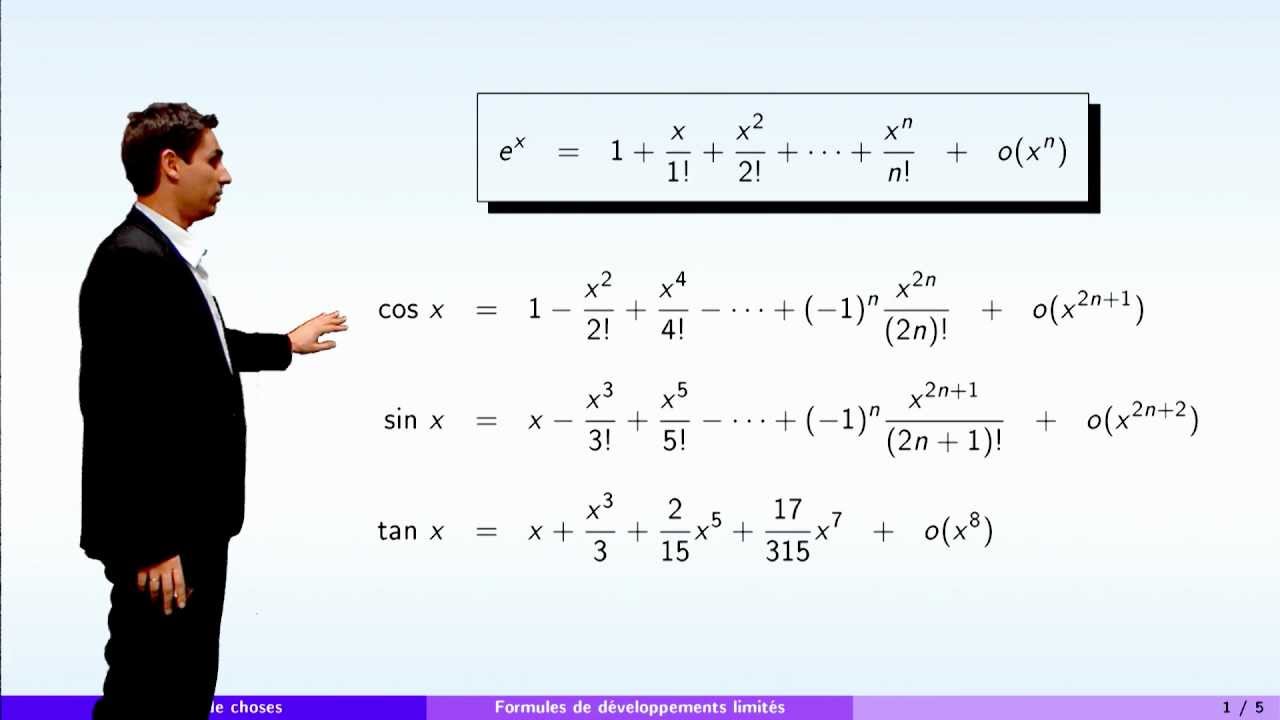
MacrosFiches.dvi. Développements limités usuels. Les développements limités ci-dessous sont valables quand. x tend vers 0. et uniquement dans ce cas. n. f(k)(0) Formule de. Taylor-Young.

Formulaires disponibles Développement limité en 1
Pour déterminer le développement limité d'une fonction f en un réel a ≠ 0 , on calcule f ( a + h) en fonction de la variable h et on cherche un éventuel développement limité de l'expression obtenue lorsque h tend vers 0. Puis on remplace h par x − a.

L1 Analyse développement limité de (1+x)^alpha YouTube
1. Let α ∈R α ∈ R. Prove that, for all x ∈ [0, 1) x ∈ [ 0, 1) we have (1 + x)α = 1 + αx 1! + α(α−1)x2 2! + ⋯ + α(α−1)⋯(α−k+1)xk k! + ⋯ ( 1 + x) α = 1 + α x 1! + α ( α − 1) x 2 2! + ⋯ + α ( α − 1) ⋯ ( α − k + 1) x k k! + ⋯. I'm not sure how to do this. Maybe Taylor's Remainder Theorem. Any hints or.
Concours PUISSANCE ALPHA 2012 Chimie (Sujet) AlloSchool
Maths L1 AnalyseDans cette vidéo on calcule le développement limité de la fonction 1/(1+x) en utilisant la formule de Taylor YoungNiveau licence scientifique.
